Non-gravitational force model vs observation: the trajectory and rotation-axis of comet 67P/Churyumov-Gerasimenko

The determination of non-gravitational forces based on precise astrometry is one of the main tools to establish the cometary character of interstellar and solar-system objects. The Rosetta mission to comet 67P/C-G provided the unique opportunity to benchmark Earth-bound estimates of non-gravitational forces with in-situ data. We determine the accuracy of the standard Marsden and Sekanina parametrization of non-gravitational forces with respect to the observed dynamics. Additionally we analyse the rotation-axis changes (orientation and period) of 67P/C-G. This comparison provides a reference case for future cometary missions and sublimation models for non-gravitational forces.

Orbit changes by non-gravitational forces
Among several thousand candidate orbits we have conducted an exhaustive search for the best-fit trajectory ofcomet 67P/C-G to pin down the magnitude and direction of the non-gravitational. Starting from estimates of the non-gravitational Marsden parameters A₁, A₂, A₃ from [1] and [2] (derived from Earth-bound observations of several apparitions of 67P/C-G), we determined an improved solution compatible with Rosetta telemetry [3]
.
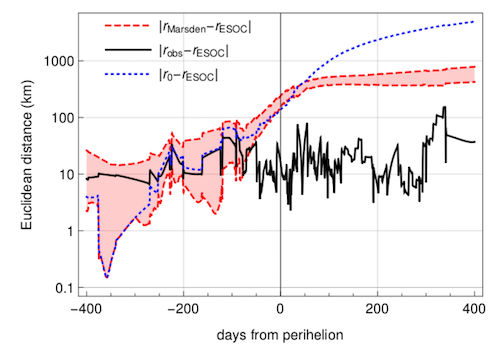
Figure 1: Error for non-gravitational force models for comet 67P/C-G [7]. The label r_ESOC denotes the (noisy) ESA provided orbit, the pink curve the best fit Marsden values, the blue curve is computed without non-gravitational forces (r₀). The reference orbit r_obs shows our best fit to the ESOC data.

Fig. 1 shows that the improved solution reduces the residual error from several hundred kilometers (Marsden parametrization) to about 20 km. This allows us to extract the magnitude anddirection of the non-gravitational acceleration (Fig. 2).

Figure 2: Total magnitude of the non-gravitational acceleration observed for 67P/C-G [7] and water production rate [8]

Comet 67P/C-G displayed a very regular activity pattern with diurnally repeating dust and gas emission [4, 5,6]. This in turn suggests a very uniform non-gravitational acceleration and smooth changes of the rotation axis.

Rotation-state changes
The rotation of comet 67P/C-G shows almost no wobbling motion and changes only by 0.5 DEG over the 2015 apparition [9]. This change is smaller than what a homogeneous emission model (i.e. Keller A model) predicts. In addition it indicates a very close alignment of the axis of inertia with the rotation axis. This requires a slightly inhomogeneous mass distribution with an increased density in the larger lobe.

Summary and Conclusions
We followed the state vector changes of the nucleus of 67P/C-G (momentum and angular momentum) in terms of a Fourier decomposition of the diurnal outgassing in the nucleus-fixed frame. Our analysis constrains the gas release and the inhomogeneity of the near surface ices. For 67P/C-G we find that the standard Marsden parametrization of the non-gravitational forces [10] can be improved considerably. No evidence for a forced precession is seen for 67P/C-G. Our methodology can be applied to other small-bodies with outgassing activity, provided that a shape and initial rotation state is known.

Acknowledgements
The work was supported by the North-German Supercomputing Alliance (HLRN). We acknowledge helpful discussions and joint work concerning the rotational state of 67P/C-G with E. Kührt, H.U. Keller, L. Jorda, andS. Hviid [9].

References
[1] Krolikowska, M. 67P/Churyumov-Gerasimenko - Potential Target for the Rosetta Mission.Acta Astronomica53, 195–209 (2003).
[2] Horizons. Asteroid & Comet SPK File Generation Request. https://ssd.jpl.nasa.gov/x/spk.html (2019).
[3] Godard, B., Budnik, F., Muñoz, P., Morley, T. & Janarthanan, V. Orbit Determination of Rosetta AroundComet 67P/Churyumov-Gerasimenko.Proceedings 25th International Symposium on Space Flight Dynam-ics–25th ISSFD, Munich, Germany(2015).
[4] Kramer, T. & Noack, M. On the origin of inner coma structures observed by rosetta during a diurnalrotation of comet 6P/Churyumov–Gerasimenko.The Astrophysical Journal823, L11–L11 (2016). DOI10.3847/2041-8205/823/1/L11.
[5] Kramer, T., Läuter, M., Rubin, M. & Altwegg, K. Seasonal changes of the volatile density in the coma andon the surface of comet 67P/Churyumov-Gerasimenko.Monthly Notices of the Royal Astronomical Society469, S20–S28 (2017). DOI 10.1093/mnras/stx866.
[6] Kramer, T., Noack, M., Baum, D., Hege, H.-C. & Heller, E. J. Dust and gas emission from cometary nuclei:The case of comet 67P/Churyumov–Gerasimenko.Advances in Physics: X3, 1404436–1404436 (2018).DOI 10.1080/23746149.2017.1404436.
[7] Kramer, T. & Läuter, M. Outgassing-induced acceleration of comet 67P/Churyumov-Gerasimenko.Astron-omy & Astrophysics630, A4 (2019). DOI 10.1051/0004-6361/201935229.
[8] Läuter, M., Kramer, T., Rubin, M. & Altwegg, K.Surface localization of gas sources on comet67P/Churyumov-Gerasimenko based on DFMS/COPS data.Monthly Notices of the Royal AstronomicalSociety483, 852–861 (2019). DOI 10.1093/mnras/sty3103.
[9] Kramer, T.et al.Comet 67P/Churyumov-Gerasimenko rotation changes derived from sublimation-inducedtorques.Astronomy & Astrophysics630, A3 (2019). DOI 10.1051/0004-6361/201834349.
[10] Marsden, B. G. Comets and Nongravitational Forces.The Astronomical Journal73, 367 (1968). DOI10.1086/110640.