Modeling Solid-State Convection in Asteroids and Meteorite Parent Bodies

Introduction: Asteroid belt observations, meteorite investigations and models converge on the paradigm of the ubiquity of melting in planetesimals. Partial melting of bodies that surpassed the melting temperature led to the weakening of the rock by the interstitial melt, facilitating solid-state convection due to a decrease of the viscosity. Further melting can produce liquid-like layers with suspended particles, i.e. magma oceans. Models^[1] indicate that ²⁶Al-heated bodies experienced differing melting degrees. In fact, transient magma oceans are likely for those that accreted early and massive (Vesta^[2]), consistent with the meteorite record^[3]. Partially molten layers can occur in the interior of undifferentiated bodies and in silicate mantles of differentiated ones. Attempts to model and to quantify the effects of convection in planetesimals remain rare. They are typically restricted to parametrized approaches in a magma ocean, neglecting convection before a magma ocean develops and after it crystallizes, and in the layers below a shallow magma ocean. However, while convection in a solid planetesimal is less likely, it may set in for little partial melt due to the weakening of solid material by the interstitial melt.

Here, the possibility of solid-state convection in planetesimals is investigated and an initial comparison of a 3D convection model with a conductive case for a Vesta-like body is presented. Implications for theoretical studies of meteorite parent bodies are discussed.

Planetesimal convection: The convective heat transport can be described with the Nusselt-Rayleigh relation with the Nusselt number Nu, the Frank-Kamenetskii parameter Θ, the Rayleigh number Ra, and parameters a, c, and β. The strength of the convection increases with Ra that depends strongly on the layer thickness and inversely on the viscosity, while the latter depends exponentially on the inverse temperature via an Arrhenius relation. If partial melting occurs, the viscosity decreases with the melt fraction χ according to η(χ)~exp(-α_ηχ) (where α_η is a constant that depends on the creep regime), e.g. by a factor of 10^-4 for χ=0.25^[4]. Further melting up to the rheologically critical melt fraction χ_RC of 0.25-0.5 marks a transition to liquid-like behavior, resulting in a magma ocean. The likelihood of convection is estimated with the (internal) Rayleigh number Ra_Q as a function of the layer thickness for different values of the viscosity (Fig. 1). In general, with decreasing viscosity and increasing layer thickness, Ra increases and convection sets in for Ra_Q≥Ra_c≈10³. An increased heat transport will result in a temperature and melt evolution that may differ from what is predicted by conventional models and have important consequences: (1) A more efficient cooling of a partially molten planetesimal. (2) Producing high amounts of melt or a magma ocean may be more difficult. (3) Convection in a partially molten mantle may sufficiently cool the core to generate a magnetic field in the absence of a magma ocean.

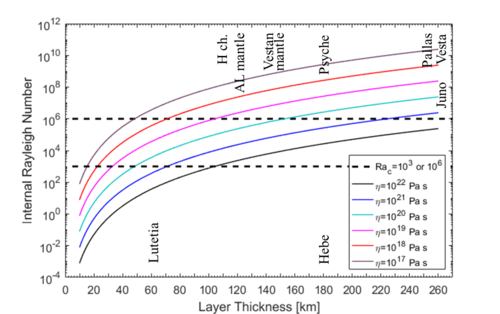
Figure 1. Ra_Q for different viscosities for a heat production at 2.1 Myr after CAIs suggest that planetesimals as small as some asteroids and H chondrite and Acapulco-Lodran parent bodies^[5,6] could convect at small degrees of melting (figure from [4]).

Vesta:  A 3D full sphere mantle convection model GAIA^[7] was used. It solves a set of differential equations based on the principle laws of conserving mass, momentum, and energy. Here, stagnant-lid regime and a free-slip boundary condition at the surface of a purely internally heated sphere were considered for a Vesta-like body with a radius of 260 km and constant material properties. A purely internal heat production matching an accretion time at three half-lives of ²⁶Al after CAIs guarantees that the melt fraction in the interior remains below χ_RC. An unddifferentiated structure was assumed for simplicity^[2].

Conductive and convective models are compared: Fig. 2 shows the temperature (top) and a combined temperature and velocity field (bottom) (top: conduction, bottom: convection). A Rayleigh number of 2×10⁶ used is consistent with η(χ)≈10¹⁹ Pa s for χ≤0.1, a layer thickness of ≥120 km, and a heat production of 3.33×10^-8 W kg^-1. Both the temperature distribution and the convection velocity differ. The convection model produces plumes that are lacking for the conductive case. The maximum temperature is higher for the conductive case showing a substantial reduction if convection is considered. This reflects effective heat dissipation by the redistribution of warmer and colder material by convective currents.

Conclusions: 3D convection modeling is needed to address abundant questions relating to planetesimal evolution. Ra estimates imply that convection is likely and should cool effectively early accreted and sufficiently large bodies. If one of these conditions is not fulfilled, it can be compensated by other factors, e.g., despite Ceres' weak present-day heat production, convection could be possible due to low-viscosity clay minerals mantle composition^[8].

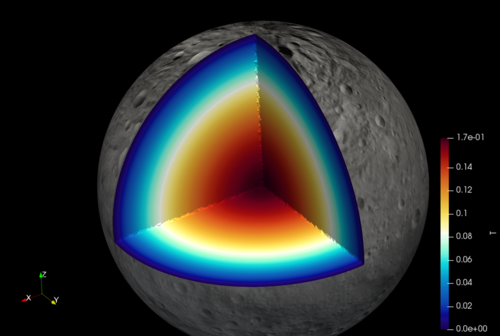
Figure 3. Temperature (top) and of temperature and velocity (bottom)^[4] of a conductive case (top) and a convective one at Ra_Q =2×10⁶ (bottom).

Efficient cooling could cause a feedback on the melting, delaying or potentially preventing the differentiation. However, convection could also help to initiate melt separation^[9] and to overcome permeability problems^[10]. Another exciting question is the possibility of convection in the mantles of differentiated bodies that may sufficiently cool the underlying cores to initiate their thermal convection and power dynamos, consistent with the remnant magnetization in basaltic meteorites^[11]. More generally, convection is relevant to studies that link models to geochemical data, building on the assumption of an “onion-shell” structure, where it will create lateral inhomogeneities and lead to different parent body properties.

References: [1] Neumann W. et al. (2012) A&A, 543, A141. [2] Neumann W. et al. (2014) EPSL, 395, 267. [3] Righter K., Drake M. J. (1997) MPS, 32, 929. [4] Neumann W. (2019) MNRAS, 490, L47. [5] Henke S. et al. (2012) A&A, 545, A135. [6] Neumann W. et al. (2018) Icarus, 311, 146. [7] Hüttig C. et al. (2013) PEPI, 220, 11. [8] Ruesch O. et al. (2019) Nat. Geosci., 12, 505. [9] Rushmer T. et al. (2000) Origin of the Earth and Moon, UAP, Tucson. [10] Ghanbarzadeh et al. (2017) PNAS, 114, 13406. [13] Weiss B. et al. (2008) Science, 322, 713.