Theoretical reference estimate for the components of the global energy balance

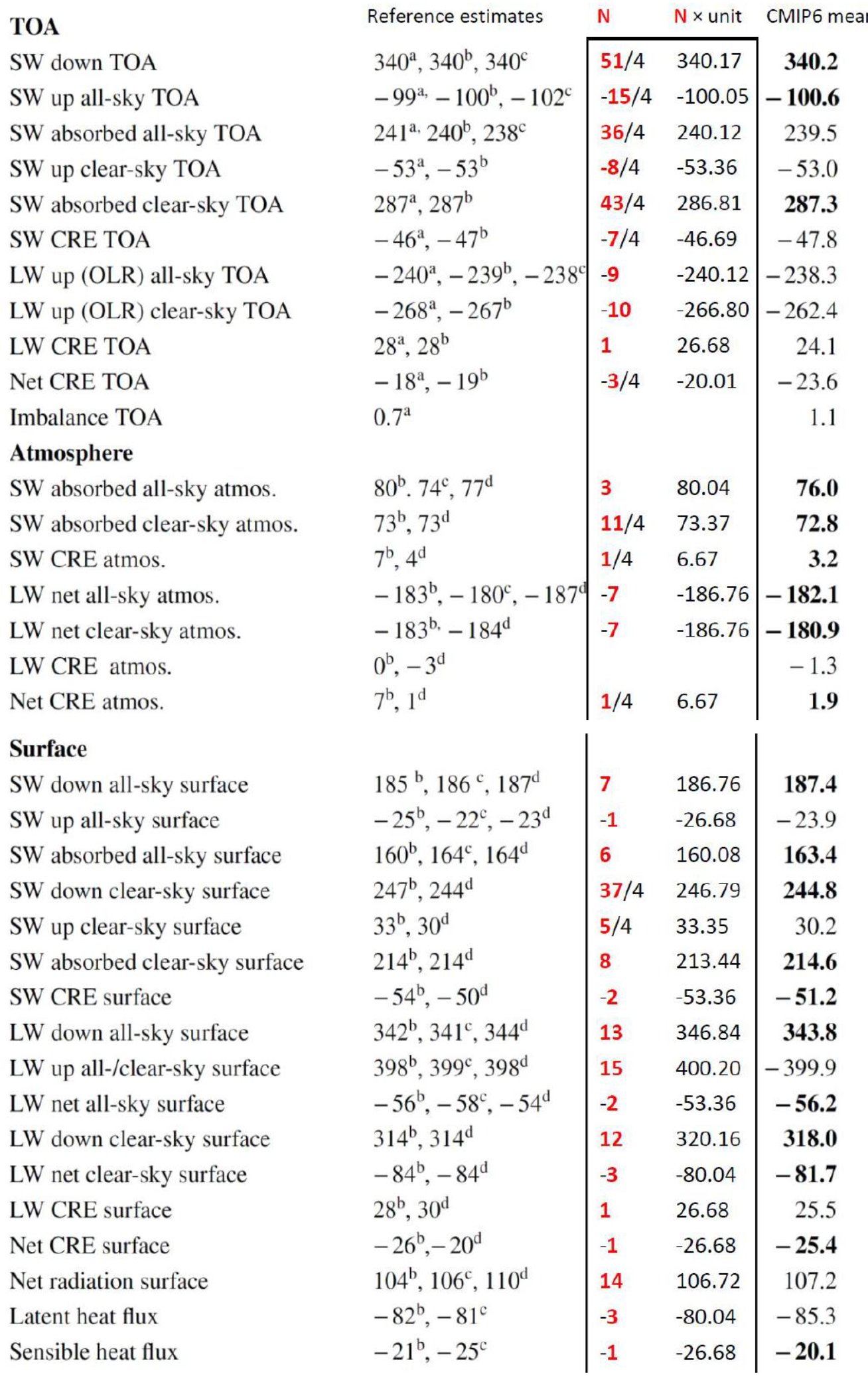
Wild (2020), and Wild and Bosilovich (2024) provide estimates of global mean energy balance components as represented in climate models and reanalyses, with reference estimates from Loeb et al. (2018), Wild et al. (2015, 2019), L’Ecuyer et al. (2015) and Kato et al. (2018). Here we add a theoretical reference estimate (TRE) based on four radiative transfer equations and geometric considerations as detailed in Zagoni (2025). The equations do not refer to the atmospheric gaseous composition or the reflective properties of the surface or clouds. The first equation is a clear-sky constraint relationship on the net radiation at the surface (R_N), following from the two-stream approximation of Schwarzschild’s (1906-Eq.11) radiative transfer equation as given in standard university textbooks on atmospheric physics and radiation (Goody, Oxford, 1964_Eq.2.115; Houghton, Cambridge, 1977_Eq.2.13; Hartmann, Academic Press 1994, Eqs. 3.51-3.54;  Ambaum, RoyalMetSoc, 2021_Eq.10.56), and in university lecture notes (Stephens 2003): R_N=OLR/2. The second equation is a clear-sky constraint relationship on the total radiation at the surface (R_T), following from the simplest greenhouse geometry (Hartmann 1994, Fig.2.3): R_T=2OLR. The third and fourth equations are all-sky versions of the first pair: R_N(all-sky)= (OLR–LWCRE)/2, and R_T(all-sky)=2OLR+LWCRE. Two decades of CERES observations (EBAF Ed4.1 April 2000–March 2022) give –2.33, –2.82, 2.71 and 2.44 [Wm^-2] deviations for the four equations, respectively, with a mean difference of 0.00. The all-sky equations are justified by an independent estimate of GEWEX within 0.1 Wm^-2 (Zagoni 2024). The solution can be given in small integer ratios relative to LWCRE as the unit flux; the best fit is 1 unit = 26.68 Wm^-2, see Table1 (highres figures and other info about TRE available at TABLELINK). Some of the most remarkable precisions are in TOA SW up all-sky (=100) and clear-sky (=53). — Li, Li, Wild and Jones (2024) provide a global radiation budget from a surface perspective from 34 CMIP6 models for 2000-2022, with differences from the TRE integer positions less than 1 Wm^-2 in SW down radiation, Thermal down Surface and the convective flux (Sensible heat + Latent heat); less than 2 Wm^-2 in Thermal up Surface; and less than 3 Wm^-2 in Reflect by surface; each within the noted ranges of uncertainty. Stackhouse et al. (2024) give Earth radiation budget at top-of-atmosphere; TRE differ from 2001-22 Climatological Mean in OLR, TSI and RSW by 0.23, 0.03 and 1.05 [Wm^-2], see details in TABLELINK in References.

 

References

Li, X., Li, Q., Wild, M. and Jones, P. (2024) An intensification of surface EEI. NatureCommE&E, https://www.nature.com/articles/s43247-024-01802-z

Stackhouse, P., et al. (2024) State of the Climate 2023, Bull. Am. Met. Soc. 105:8, https://journals.ametsoc.org/view/journals/bams/105/8/2024BAMSStateoftheClimate.1.xml

Stephens, G. (2003) Colorado_State_University_AT622_Section 6_Eqs. (6.10a)-(6.10b), Example 6.3, Fig. 6.3a, https://reef.atmos.colostate.edu/~odell/AT622/stephens_notes/AT622_section06.pdf

Wild, M. (2020) The global energy balance as represented in CMIP6 climate models. Climate Dynamics 55:553–577, https://doi.org/10.1007/s00382-020-05282-7

Wild, M., Bosilovich, M. (2024) The global energy balance as represented in reanalyses. Surv Geophys, https://link.springer.com/article/10.1007/s10712-024-09861-9

Zagoni, M. (2024) Modeling and Observing Global Energy and Water Cycles by GEWEX. AGU Fall Meeting, https://agu.confex.com/agu/agu24/meetingapp.cgi/Paper/1535956

Zagoni, M. (2025) Trenberth’s Greenhouse Geometry. AMS Annual Meeting, https://ams.confex.com/ams/105ANNUAL/meetingapp.cgi/Paper/445222  see also the updated Supplementary Material video: https://www.earthenergyflows.com/Zagoni-EGU2024-Trenberths-Greenhouse-Geometry_Full-v03-480.mp4

TABLELINK: https://earthenergyflows.com/TRE20.pdf