Conditions for Axisymmetric Viscous Overstability in Self-gravitating rings

 

Saturn's rings exhibit a phenomenal wealth of structures covering a vast range of length scales (Porco et al. 2005). On the sub-kilometer scale, various instruments aboard the Cassini mission revealed the presence of quasi-periodic radial density variations at several locations of Saturn’s B and inner-A ring, where optical depth ∼1–2 (Colwell et al. 2007; Thomson et al. 2007; Hedman et al. 2007). To date, axisymmetric viscous overstability offers the most plausible explanation for these short-scale axisymmetric structures.

The emergence of viscous overstability was directly demonstrated for the first time in fully self-gravitating 3D N-body simulations, once the optical depth τ≥1. Otherwise, in the absence of self-gravity no overstability was seen until τ∼4. Further analysis showed that the ring’s vertical self-gravity is crucial in promoting the overstable behaviour at optical depths close to unity (Salo et al. 2001).

So far, the theoretical understanding of overstable ring systems relies on analytical results based on approximate treatments of ring self-gravity that omit the emergence of self-gravity wakes. The interplay between these two mechanisms, self-gravity and overstability, is still not well understood. The above mentioned self-consistent numerical experiments in Salo et al. (2001) indicated that self-gravity wakes and overstable oscillations can coexist, although only if the self-gravity is not too strong. More recently, Ballouz et al. (2017) confirmed this and moreover demonstrated that overstability can tolerate substantially stronger self-gravity if friction and sticking forces between particles are included in simulations.

We present a systematic study on the onset of viscous overstability in self-gravitating dense rings, in which we address numerically the factors that determine its occurrence: optical depth, particle elasticity and gravity-related parameters r_h and/or Ω_z/Ω. For this purpose we use our novel adaptation of the open-access code REBOUND (Rein & Liu 2012). Our version uses soft-sphere particle collisions while retaining the originally implemented Barnes-Hut tree algorithm for a self-consistent calculation of particle-particle gravity. Some details concerning this new version of the source code are discussed, as it will be publicly released in the near future.

We find that weak self-gravity promotes overstability whereas strong self-gravity, with prominent wake structure, weakens the overstable pattern. This strong self-gravity regime corresponds to large optical depths at intermediate r_h, or to moderate optical depths at high r_h. While the dependence on r_h is known from earlier simulations, the suppression at high optical depths is a new finding. Both cases are illustrated in Figures 1 and 2.

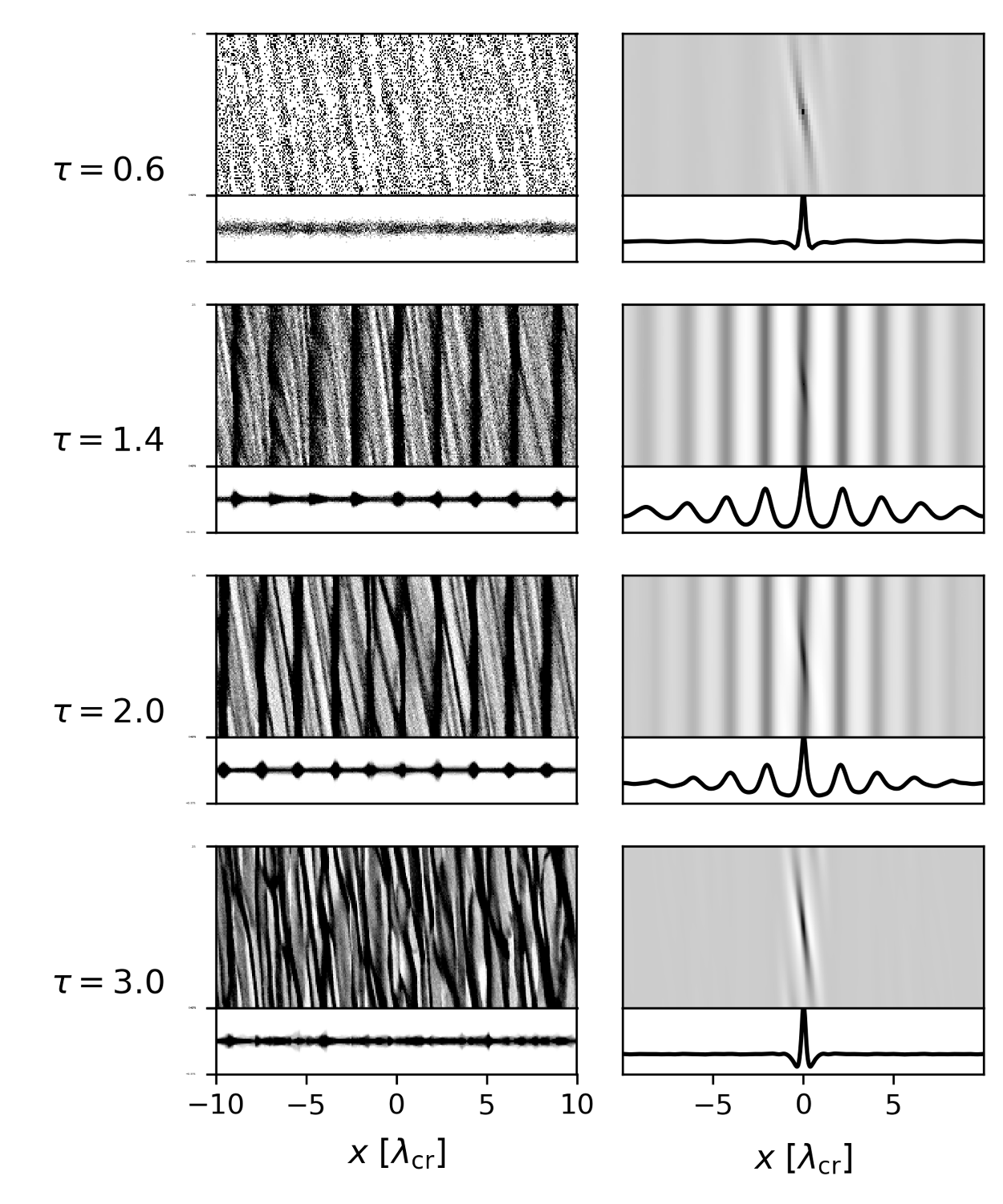
Figure 1. Self-gravitating local simulations with different optical depths, for fixed r_h=0.61 and ε=0.1. The dimensions of the simulation box are 20λ_cr × 5λ_cr. Left column: Snapshot of the x-y (top) and x-z (bottom) particle distribution at 300 orbital periods. Notice that in the zx-projection the vertical scale is stretched by a factor 5. Right column: 2D autocorrelation function ζ_2D(x,y) together with the corresponding 1D radial cut along the abscissa, averaged over 150–300 orbital periods. Grey scale extends from 0.9 to 1.4 times the mean value, while ζ_2D(x,0) is displayed in the range 0.85–1.35.

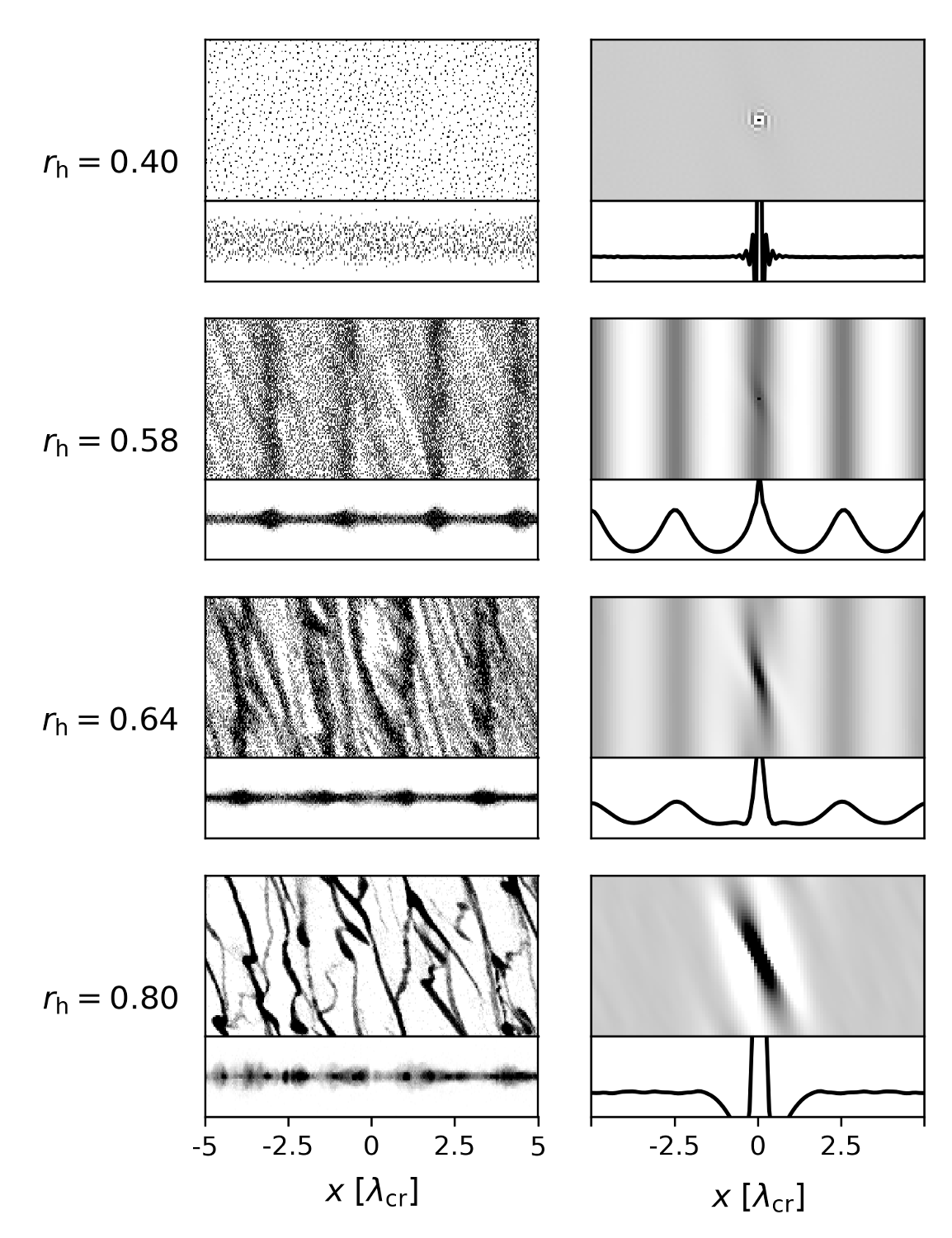
Figure 2. Self-gravitating local simulations with τ=1.0 and ε=0.1. The dimensions of the simulation box are 10λ_cr × 5λ_cr. Left column: Snapshot at 300 orbits. Note that the xz-projection the vertical scale is stretched by a factor of 3 compared to the radial. Right column: 2D autocorrelation function ζ_2D(x,y) together with the corresponding 1D radial cut along the abscissa, averaged over 150–300 orbital periods. Grey scale and ζ_2D(x,0) ranges are the same as in Figure 1.

 

To investigate the separate role of ring particles’ mutual self-gravity components in the promotion/suppression of viscous overstability we conduct a new type of hybrid experiments which combine a self-consistently calculated planar self-gravity with a separately adjusted vertical gravity field. Whereas in the case of fully self-consistent self-gravity the overstability is limited to r_h=0.5–0.6, in hybrid method this regime can be extended using larger Ω_z/Ω (see Figure 3); in this respect the method mimics the effects of including particle-particle surface forces done in Ballouz et al. (2017).

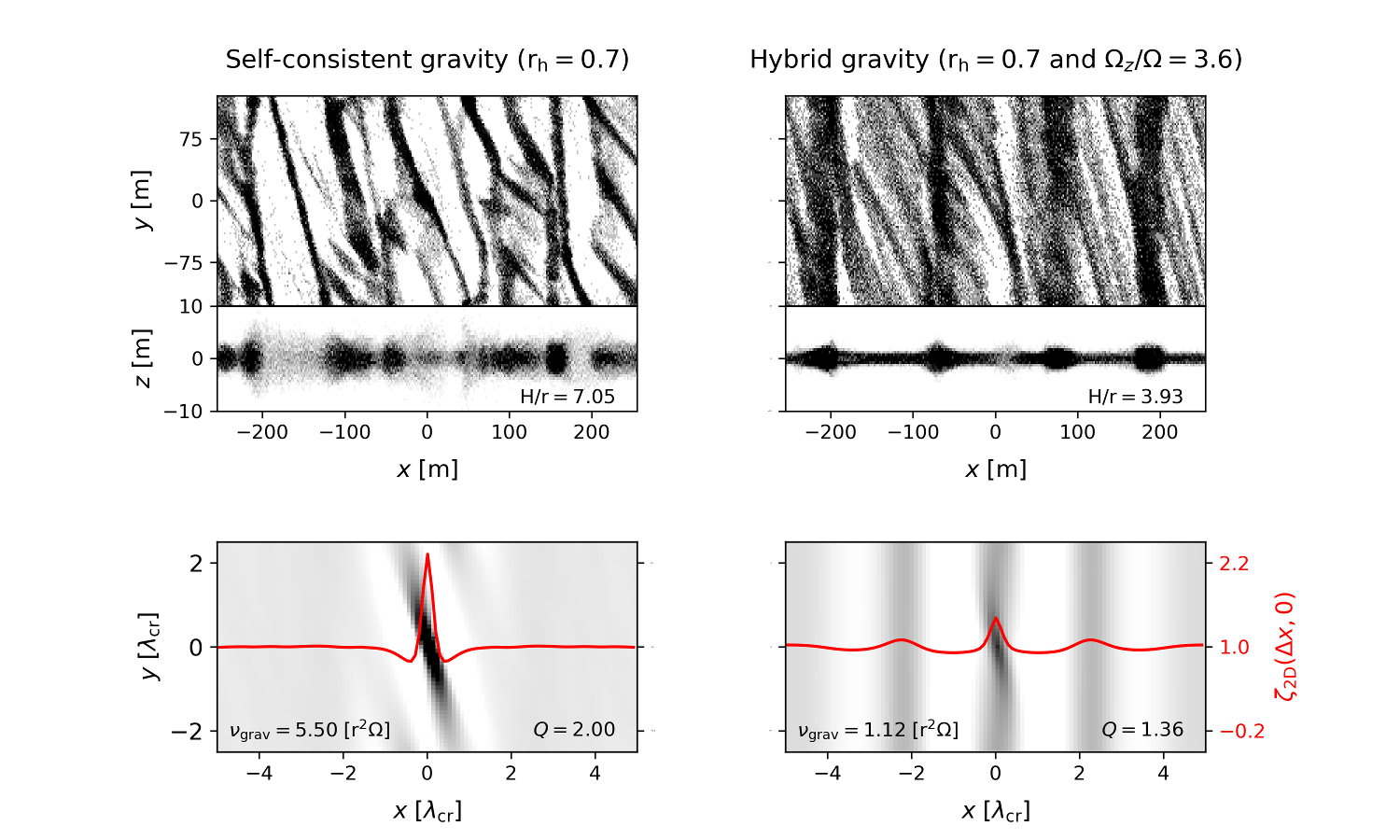
Figure 3. Comparative example between self-consistent (left) and hybrid (right) self-gravity simulations at r_h=0.70 with τ=1.0 and ε=0.1. The vertical frequency enhancement use for hybrid method is Ω_z/Ω=3.6. Some physical quantities like Toomre parameter Q (Toomre 1964), ring vertical thickness in terms of particle radius H/r, and the gravitational viscosity component ν_grav are provided inside the panels. Top: Snapshot after 300 orbital periods. Bottom: 2D autocorrelation function averaged over 150–300 orbital periods together with the corresponding radial cut at y=0.

 

Finally, we derive a composite criterion for the onset of overstability valid even in the regime of strong gravity wakes and framed in terms of collective properties of the system: the central-plane filling factor and the ratio of gravitational and collisional viscosities. The combined semi-empirical condition reads:

FF(0) > FF(0)_cr(r_h) and ν_grav / ν_coll ≤ 0.3,

where FF(0)_cr decreases monotonically when the strength of ring-plane self-gravity is increased, from ∼0.45 for the non-gravitating case to ∼0.3 at r_h=0.76. In the limit where gravity wakes are omitted, this criterion agrees with that found by the kinetic theory analysis of the linear stability in Latter & Ogilvie (2008).

 

References:

Ballouz R.-L., Richardson D. C., Morishima R., 2017,AJ, 153, 146

Colwell J. E., Esposito L. W., Sremčević M., Stewart G. R., McClintockW. E., 2007,Icarus,190, 127

Hedman M. M., Nicholson P. D., Salo H., Wallis B. D., Buratti B. J., BainesK. H., Brown R. H., Clark R. N., 2007,AJ, 133, 2624

Latter H. N., Ogilvie G. I., 2008,Icarus,195, 725

Porco C. C., et al., 2005,Science,307, 1226

Rein H., Liu S. F., 2012,A&A,537, A128

Salo H., Schmidt J., Spahn F., 2001,Icarus,153, 295

Thomson F. S., Marouf E. A., Tyler G. L., French R. G., Rappoport N. J.,2007,Geophys. Res. Lett.,34, L24203

Toomre A., 1964,ApJ,139, 1217