Statistical clustering analysis of NEOs to find correlations with spectral classes
- 1INAF - Osservatorio Astronomico di Roma, Monte Porzio Catone, Italy (prasanna.deshapriya@inaf.it)
- 2Department of Earth, Atmospheric and Planetary Sciences, Purdue University, West Lafayette, IN 47907, USA
- 3Department of Physics, Physicum D312, P.O. Box 64 (G. Hällströmin katu 2a), FI-00014 University of Helsinki, FINLAND
- 4Asteroid Engineering Laboratory, Space Systems, Luleå University of Technology, Box 848 (Rymdcampus, E10), S-981 28 Kiruna, SWEDEN
- 5LESIA‐Observatoire de Paris, Université PSL, CNRS, Sorbonne Université, Université de Paris, 5 place Jules Janssen, 92195 Meudon, France
- 6Agenzia Spaziale Italiana, Via del Politecnico 1, 00133, Rome, Italy
Introduction: Study of near-Earth objects (NEOs) is crucial to better understand the origin, formation and the evolution of the solar system. In particular, compositional, morphological and orbital characterisation of NEOs sheds light on the delivery of water and organics [1,2,3] to the prebiotic Earth, while ironically, some NEOs could be potential hazards for life on Earth [4]. In this context, we apply the G-mode multivariate statistical clustering method [5,6,7] on the orbital parameters of the currently available NEOs population, to probe potential associations with their spectral classification [8,9,10].
Data and Methods: G-mode method leads to an automatic statistical clustering of a sample containing N objects (NEOs in this case), described by M variables with the only control imposed by the user being the confidence level q1, expressed in terms of σ. The objects that cannot be associated to a cluster are separated and considered as outliers. We apply G-mode multivariate statistical clustering analysis to variables defining NEOs to determine clustering of objects.
Our sample consists of 14132 NEOs, filtered based on their orbital uncertainty. Our input parameters to the G-mode method are twofold. First, in approach 1 (A1) we used three variables and their uncertainties as inputs: inclination (i), eccentricity (e) and semi-major axis (a) of the orbit. In approach 2 (A2) we used six inputs by including the argument of perihelion (ω), Tisserand parameter with respect to Jupiter (TJ) and the absolute magnitude (H) and their respective uncertainties in addition to those used in A1.
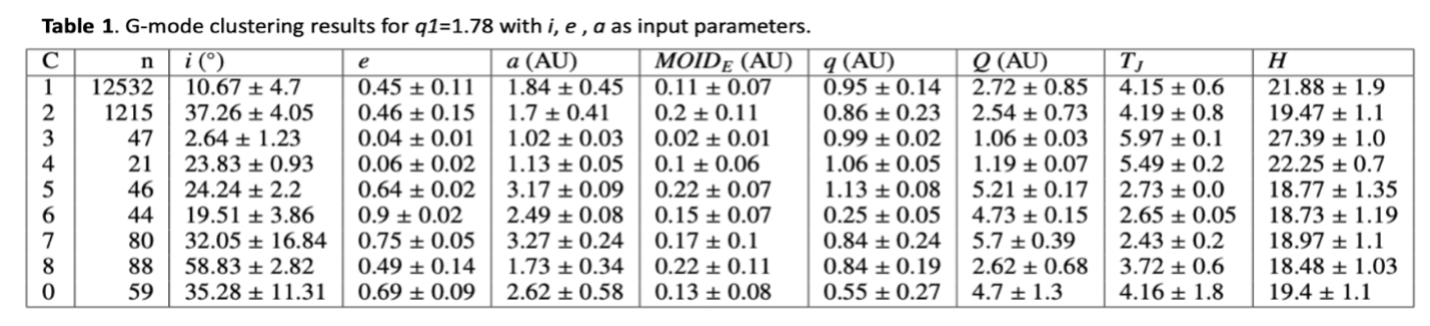
Results: In A1, we obtained only one cluster at q1=3σ (accurate classification probability of 99.7%), which meant that the NEOs in this cluster are homogeneous in terms of the three orbital elements used. As we gradually lowered the value of q1, hence the confidence level, we noticed that more clusters got seeded and populated. We report in Table 1, mean parameter values with the median absolute deviation for 8 clusters obtained at q1=1.78σ. In addition to reporting the statistics of obtained clusters, we have also reported the outlier group of NEOs in the row indexed by 0. Among the reported clusters, cluster 1 (C1) corresponds to the background population of NEOs as their statistics are comparable to those of all hitherto discovered NEOs. C2 represents NEOs with relatively moderate inclination. C3, despite having relatively small number of NEOs, is constrained by well-behaved low-inclined, quasi-circular Earth-like orbits owing to their very low median absolute deviations. The very low mean H implies that these NEOs are relatively smaller with a distribution peak in the range of 5-15 meters of diameter.
C5, C6 and C7 are of particular interest, for they could be associated with Jupiter-family cometary nuclei (2 < TJ < 3) as per their mean TJ. As such, the objects in these three clusters could potentially be extinct cometary nuclei. These clusters also have relatively higher eccentricities, akin to cometary orbits. We have checked available taxonomic classifications of NEOs [11,12,13] in the literature to get an insight into the composition of the objects. Although taxonomic classifications are not available for the majority of members in the clusters, we find that (i) C5 contains 3 C-types, 2 D-types and 0 S-types (ii) C6 contains 3 D-types and 4 S-types (iii) C7 contains 2 B-types, 3 C-types, 2 D-types and 1 S-type. Therefore we argue that C5 and C7 can be associated to primitive carbonaceous compositions while C6 can be associated to both carbonaceous and silicate-dominated compositions.
To get more insights about the obtained G-mode clusters and further test these results, we applied a dynamic model to find their potential escape regions in the main belt [14]. This dynamical model, the Granvik model hereafter, traces with scrutiny the escape paths of objects from the main-belt to near-Earth space and the model accounts for the Yarkovsky effect [15]. Given the orbital elements and absolute magnitude of an NEO, it resolves a probability distribution function, built up of seven discrete values for seven escape regions. The results obtained with the Granvik model are shown in Fig. 1 for the clusters obtained for the G-mode run at q1=1.78σ in A1.
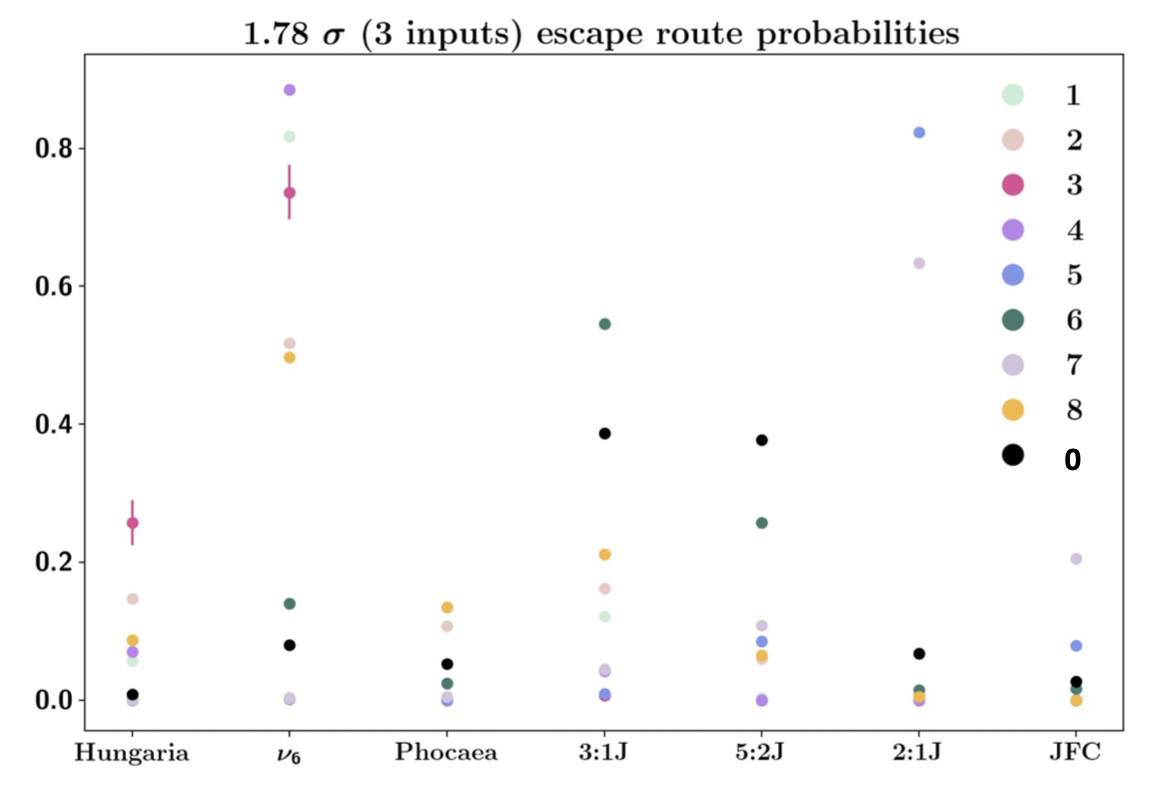
Fig. 1. Probability distributions for the G-mode clusters obtained at 1.78σ for A1 to emanate from any of the seven escape regions.
These results imply that most of the clusters have higher probability to emanate via ν6 secular resonance, known to be the most dominant escape path from the main-belt to the near-Earth space [16]. We observe that C5 and C7 have significant probabilities to emanate from outer-belt sources such as 2:1 mean motion resonance of Jupiter and JFCs. Thus, our dynamic modelling reinforces and corroborates existing results, pointing towards a primitive origin for the NEOs in these two clusters.
Similiary, we will present the results of A2 and discuss their implications.
Acknowledgements: We acknowledge the financial support from Agenzia Spaziale Italiana (ASI, contract No. 2017-37-H.0 CUP F82F17000630005) and funding from the European Union’s Horizon 2020 research and innovation programme under grant agreement No. 870403.
References:
[1] Marty, B., Guillaume, A. et al. 2016, Earth and Planetary Science Letters, Volume 441,Pages 91-102
[2] Altwegg, K, Balsiger, H, Bar-Nun, A. et al. 2015, Science, Vol. 347,6220,1261952
[3] Ehrenfreund, P. & Sephton 2006, Faraday Discuss., The Royal Society of Chemistry,133,277-288
[4]Perna, D., Barucci M.A., Fulchignoni M. 2013, Astronomy and Astrophysics Review,Vol.21,65
[5] Barucci, M.A., Capria, M.T., Coradini, A. et al. 1987,Icarus,72,304
[6] Gavrishin, A.I., et al. 1992, Earth, Moon and Planets,59,141-152
[7] Barucci, M.A., Belskaya, I., Fulchignoni, M. et al. 2005,AJ130,1291
[8] Bus, S.J., & Binzel, R.P. 2002, Icarus,158,146
[9] DeMeo, F.E., Binzel, R. P., Slivan, S.M. & Bus, S. J. 2009,Icarus,202,160-180
[10] DeMeo, F.E., Alexander, C.M.O., Walsh, K.J.et al. 2015, Asteroids IV,13-41
[11] Perna, D., Barucci,M.A. et al. 2018, Planetary and Space Science,157,82-95
[12] Devogèle, M., Moskovitz, N. et al. 2019, The Astronomical Journal, American Astronomical Society,158,196
[13] Ieva, S., Dotto, E. et al. 2020,A&A,644,A23
[14] Granvik, M., Morbidelli, A., Jedicke, R., et al. 2018,Icarus,312,181
[15] Vokrouhlický, D., Bottke, W. F., Chesley, S.R., Scheeres, D.J., & Statler, T.S. 2015, in Asteroids IV,509–531
[16] Bottke, W.F., Morbidelli,A., Jedicke,R., et al. 2002,Icarus,156,399
How to cite: Deshapriya, J. D. P., Perna, D., Bott, N., Hasselmann, P. H., Granvik, M., Dotto, E., Fulchignoni, M., Giunta, A., Perozzi, E., Ieva, S., Petropoulou, V., and Mazzotta-Epifani, E.: Statistical clustering analysis of NEOs to find correlations with spectral classes, Europlanet Science Congress 2022, Granada, Spain, 18–23 Sep 2022, EPSC2022-698, https://doi.org/10.5194/epsc2022-698, 2022.