Exploring Enceladus: Internal Structure Models and Moment of Inertia

Introduction

Enceladus, Saturn's sixth-largest moon, with a radius of 252.0±0.2 km [1] was observed by NASA’s Cassini spacecraft in 2005. During its close flybys, Cassini’s imaging science subsystem captured images revealing a region of intense geological activity near Enceladus´ South Pole [2] where it was found to be the source of fine icy particles that form Saturn’s E ring [3]. This suggests the existence of a subsurface water ocean [2].

Enceladus´ density of 1608±5 kg/m³ and its icy surface [2] indicates an ice-rich bulk composition. Additionally, the moment of inertia (MOI) coefficient, ranging from 0.33-0.34 with a confidence level linked to the 2σ range, suggests a differentiated body [4] with a rocky core beneath an H₂O mantle [5].

Our current investigation focuses on exploring various internal structures for Enceladus and their corresponding MOI coefficients to determine which internal structure aligns most closely with the MOI coefficient obtained from gravity field data.

Methodology

Several researchers have explored Enceladus's thermal and internal structure. This study builds upon the work of Neumann and Kruse [5], which examined the thermal evolution and differentiation of Enceladus heated by radionuclides and tidal dissipation.

The Neumann-Kruse model incorporates wet olivine, dry olivine, and antigorite for the core's rock rheology. This results in a differentiated Enceladus structure with a core radius of ≈185–205 km, a porous core layer of ≈2–80 km, a subsurface ocean of ≈10–27 km, and an ice-rock crust layer of ≈30–40 km.

Additionally, we adjusted Neumann-Kruse models to incorporate the subduction of an undifferentiated ice-rock crust and the settling of its rock fraction atop the core. This resulted in changes in crust density and an enlarged core radius. Various suggested crust densities were examined: 850 kg/m³ [1], 918 kg/m³, and 925 kg/m³ [6,7].

We calculated MOI for each of the Neumann-Kruse models and the adjusted models derived in this study using equation (1), where ρ(r) represents the density as a function of the radial coordinate r, and θ and φ denote the polar and azimuth angles, respectively:

(1)

The MOI coefficient is computed using:

(2) 

where M represents Enceladus' total mass, and R is its radius.

Results

Figure 1 shows the MOI coefficients calculated for differentiated Neumann-Kruse models and adjusted models for a differentiated crust with a crust density of 850 kg/m³ [1], 918 kg/m³, and 925 kg/m³ [6,7] for both dry and wet olivine core rheologies.

The results show that models without crust subduction have the lowest agreement with the derived MOI from the gravity field by [4]. However, when accounting for undifferentiated crust subduction resulting in an icy crust with a density of 925 kg/m³, most models align within the accepted range of MOI coefficients. Additionally, our findings suggest that models with a higher crust density require a higher core density, while those with a lower crust density should have a lower core density to fall within the accepted range of MOI coefficients.

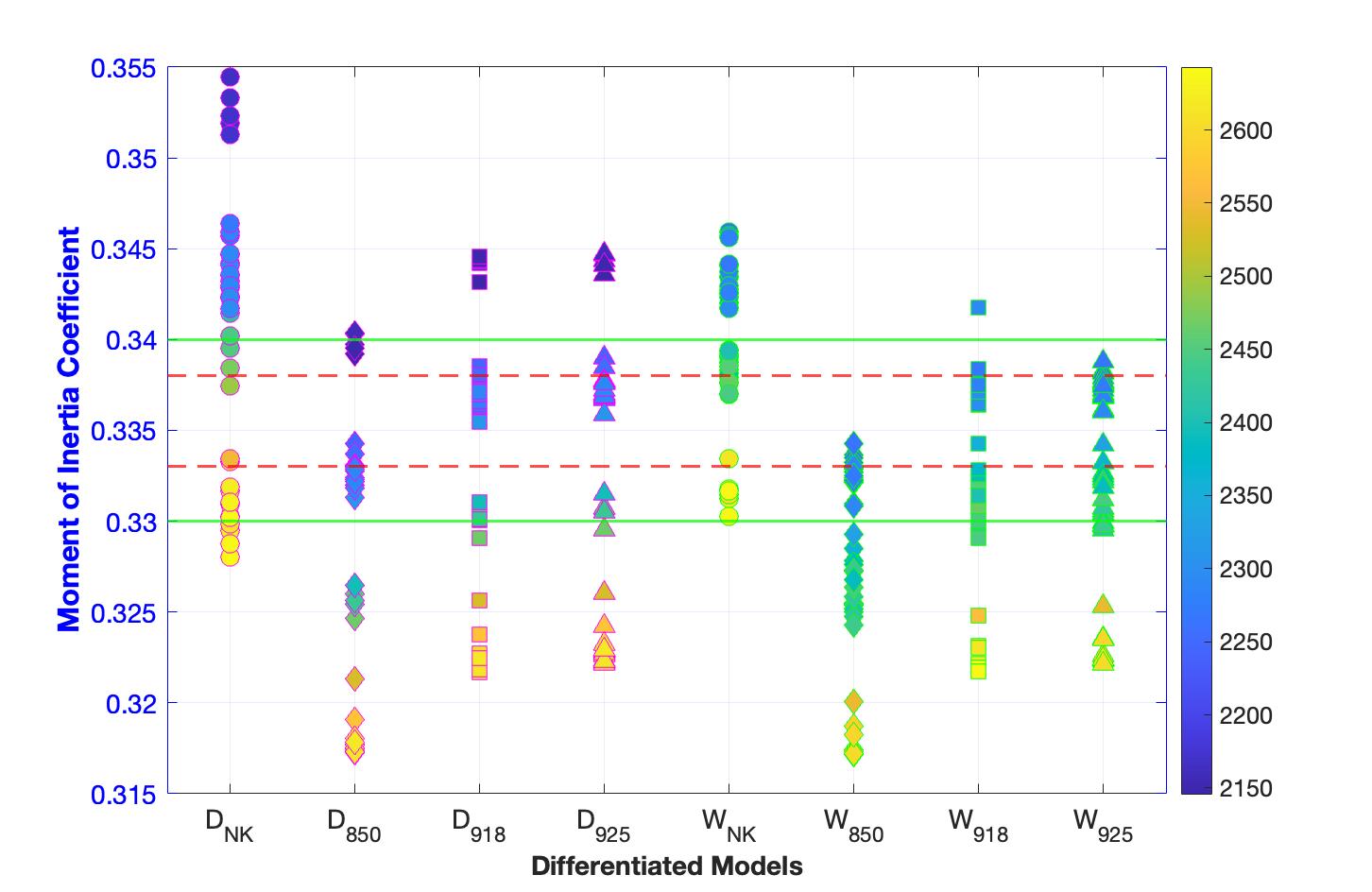
Figure 1: Results for the moment of inertia coefficient."D" and "W" denote dry and wet olivine core rheologies. "NK" signifies a crust density of 1609 kg/m³ from Neumann-Kruse models, while subscripts "850," "918," and "925" represent crust densities of 850 kg/m³, 918 kg/m³, and 925 kg/m³, respectively. The colorbar displays core densities ranging from 2150-2633 kg/m³. The solid green line marks a MOI coefficient range of 0.33-0.34, matching the 2σ range confidence level, while the red line represents a range of 0.333-0.338, matching the 1σ range confidence level [4].

When examining the radius of the outer porous core under the condition that the MOI falls within the range of 0.33-0.34 [4], the thickness of this outer porous core varies depending on the type of olivine core rheology. For dry olivine, the outer porous core's thickness ranges from ≈3-73 km, with ≈60% of models under 10 km thick and ≈80% below 20 km. Conversely, for wet olivine, the range is ≈2-80 km, ≈50% of models show a porous outer core under 10 km thick, while ≈65% are below 20 km.

Conclusions

Our study builds upon the differentiated internal structure models proposed by [5], initially based on a rock-ice crust model. We further refined these models by incorporating a differentiated crust with varying densities. Our analysis indicates that most models, with a crust density of 925 kg/m³, align with the MOI range of 0.333-0.338 [4]. This trend persists regardless of whether the core rheology is dry or wet olivine. As expected, lower crust densities result in lower average MOI coefficients. Overall, approximately 54% of the models fall within the accepted 2σ range of MOI, suggesting their compatibility with observed gravity data.

Acknowledgment

This work was supported by the Berlin University Alliance (BUA), by the Deutsche Forschungsgemeinschaft (DFG), and by the International Space Science Institute (ISSI) in Bern and Beijing, through ISSI/ISSI-BJ International Team project “Timing and Processes of Planetesimal Formation and Evolution”. 

References

[1] P.C. Thomas. et al., Icarus 264, 37 (2016).

[2] C.C. Porco. et al., Science 311, 1393 (2006).

[3] F. Spahn. et al., Science 311, 1416 (2006).

[4] L. Iess. et al., Science 344, 78 (2014).

[5] W. Neumann and A. Kruse, The Astrophysical Journal 882, 47 (2019).

[6] W.B. McKinnon, Geophysical Research Letters 42, 2137 (2015).

[7] D.J. Hemingway and T. Mittal, Icarus 332, 111 (2019).