The influence of tides on catastrophic disruptions of close-in planetary satellites

Introduction  Impact processes play a dominant role in the evolution of the solar system at nearly all size scales, from planet formation all the way to micrometeorite impacts on small bodies. The outcomes of collisions are often parameterized by Q, which is the specific impact energy. The parameter  Q^*_D denotes the catastrophic disruption threshold, which is defined as the specific energy required disperse half of the total mass involved in the collision, leaving remaining mass in the largest remnant. Many studies have attempted to determine  Q^*_D  numerically, demonstrating that it is a complicated function of the target’s physical and material properties (size, density, strength, internal structure, etc.) and impact conditions (impactor size, velocity, angle, etc.) (e.g., Benz and Asphaug 1999; Leinhardt and Stewart 2012; Jutzi 2015; Ballouz et al. 2015; Raducan et al. 2024, among others). Here, we introduce the concept of  Q^*_TD, which is the catastrophic disruption threshold for a natural satellite, accounting for tides from the central body. 
        We will present a suite of hundreds of simulations varying the impact conditions to derive a scaling relationship between the mass of the largest remnant, M_lr, and  Q^*_TD . We will show that the influence of an external tidal potential plays an important role in determining both the mass of the largest remnant and the size-frequency distribution of ejected fragments, even for targets well outside the primary’s Roche limit. Finally, we discuss the implications for this modified impact scaling law for small satellites such as Phobos, Deimos, and the inner satellites of the gas and ice giants.

Methods Most previous studies considered only non-rotating targets in inertial space, although pre-impact rotation can enhance mass loss (Ballouz et al. 2014) and the role of tides is known to play a significant role in some scenarios (Hyodo and Ohtsuki 2014). Accounting for tides in collisional disruptions means there are many more free parameters as the problem is no longer spherically symmetric. To keep the problem computationally feasible, we consider a target with a single set of physical and material properties. Then, we vary the impactor size, velocity, impact angle, impact direction, and the orbital distance of the target. We only consider relatively low-speed impacts (v ≪ 1 km s⁻¹), so both the collision and subsequent gravitational accumulation are modeled using pkdgrav, a N-body code that includes particle self-gravity and handles particle contacts using the soft-sphere discrete element method (Richardson et al. 2000; Schwartz et al. 2012; Zhang et al. 2017).

Our simulations consider a spherical target, consisting of  randomly arranged particles with a size-frequency distribution following a truncated power law with an index of -3. The particles are cohesionless and have frictional angle of . Particles have a density of 2 g cm^-3, which gives the target a bulk density and radius of ρ_bulk ~1.35 g cm and R~10 km, respectively. The target has a surface escape velocity of v_esc ~9 m s⁻¹. The target is placed on a circular orbit around a Mars-mass primary at varying semimajor axes and allowed to settle into an equilibrium before the impact is performed. These simulations assume the target to be tidally locked to the primary, so the target’s spin rate varies along with its orbital distance. The target is slightly smaller in size and less dense than Phobos, however the results can be generalized to any small satellite around a planet.

Preliminary Results We show an example of a head-on collision with an impactor M_imp = 0.1M_targ at an impact velocity of v = 10v_esc ~ 90 m s⁻¹. Snapshots from this simulation at three orbital semimajor axes are shown in Fig. 1. These three semimajor axes correspond to aorb = [1.31, 1.44, 1.97]R_RRL, where R_RRL is the radius of the target’s rigid-body Roche limit. We see a sharp transition between the two innermost cases. When a_orb = 1.31R_RRL, the body first undergoes a catastrophic collision but the disturbs the satellite enough causing to to undergo a complete tidal disruption despite it technically being outside the Roche limit. When a_orb is increased slightly to 1.45R_RRL, the body is safe from disruption and the mass of the largest remnant is M_lr ~0.61M_tot, where M_tot is the combined mass of the target and impactor. As the orbital distance is increased further to a_orb = 1.97R_RRL, the target is able to retain more mass. We attribute this to two factors. Since the target starts in a tidally-locked configuration, a larger a_orb means a slower rotation and therefore a slightly weaker surface gravity. The second, and more important factor, is that the Hill sphere increases with a_orb, as does the escape velocity required to reach the Hill sphere, so less ejecta is able able to escape onto planetocentric orbits for the same impact.

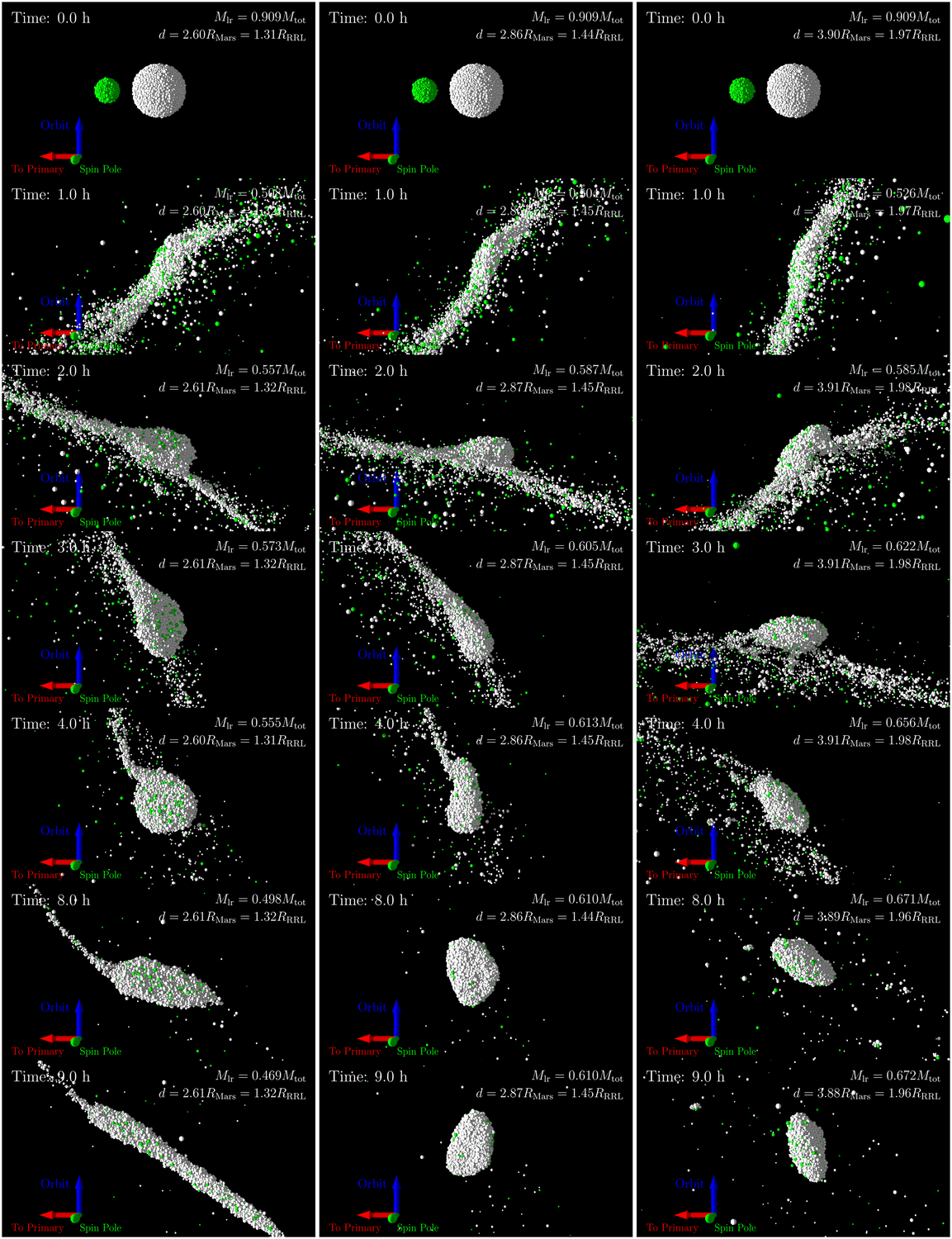
Figure 1: Snapshots from the first 9 hours of three simulations with the same impact conditions at different orbital distances. The impactor is shown in green, traveling from left to right and the target is in white. From left to right, the semimajor axis of the target’s circumplanetary orbit corresponds to 1.31, 1.44 and
1.97 times the rigid-body Roche limit (R_RRL).

Acknowledgments  H.A. was supported by the French government, through the UCA J.E.D.I. Investments in the Future project managed by the National Research Agency (ANR) with the reference number ANR- 15-IDEX-01. P.M. acknowledges funding support from CNES.

References
Ballouz, R.-L. et al. ApJ 789, 158 (2014).
Ballouz, R.-L. et al. Planet. Space Sci. 107, 29–35 (2015).
Benz, W. & Asphaug, E. Icarus 142, 5–20 (1999).
Hyodo, R. & Ohtsuki, K. ApJ 787, 56 (2014).
Jutzi, M. Planetary and Space Science 107, 3–9 (2015).
Leinhardt, Z. M. & Stewart, S. T. ApJ 745, 79 (2012).
Raducan, S. D. et al. PSJ 5, 79 (2024).
Richardson, D. C. et al. Icarus 143, 45–59 (2000).
Schwartz, S. R., Richardson, D. C. & Michel, P. Granular Matter 14, 363–380 (2012).
Zhang, Y. et al. Icarus 294, 98–123 (2017).