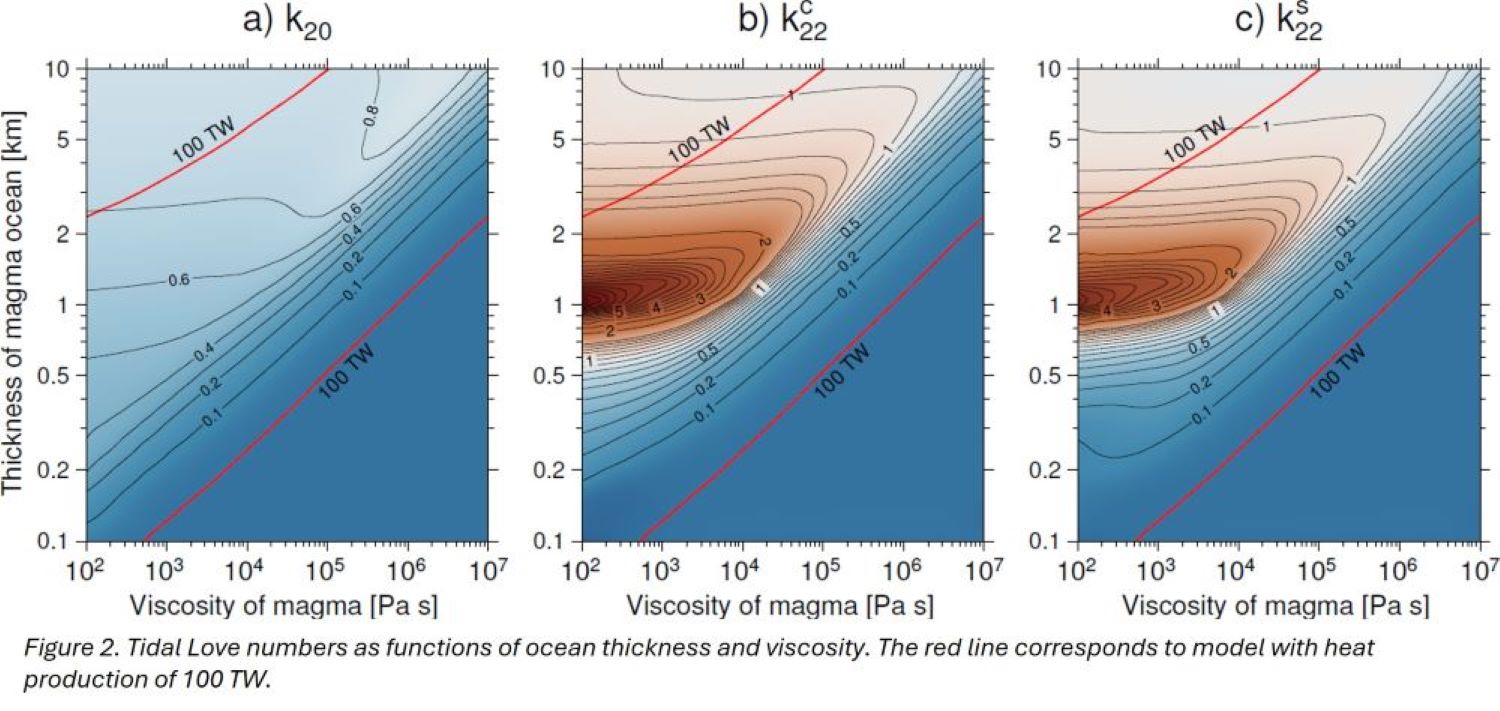
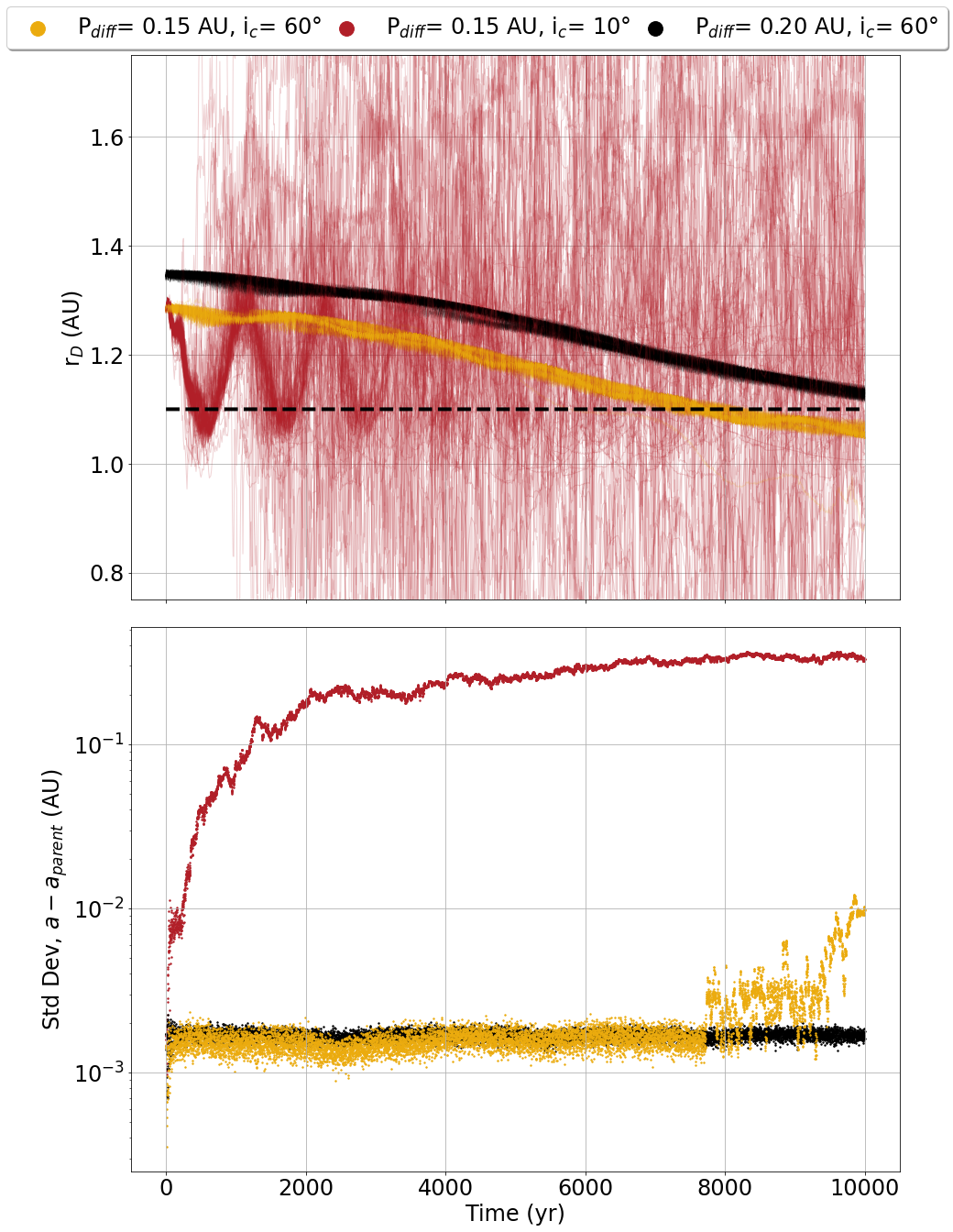
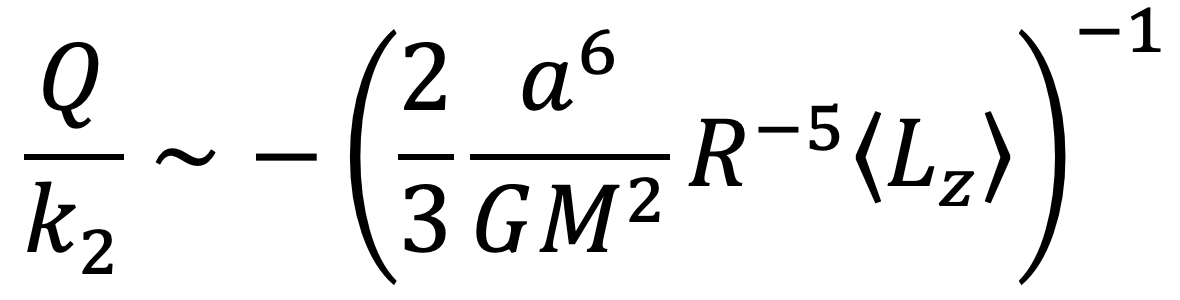TP4
Planetary Dynamics: Shape, Gravity, Orbit, Tides, and Rotation from Observations and Models
Co-organized by SB
Convener:
Alexander Stark
|
Co-conveners:
Bart Root,
Marie Yseboodt,
Anton Ermakov,
Haifeng Xiao,
Michaela Walterova
Session assets
Planets & Moons
08:30–08:40
|
EPSC2024-1144
|
On-site presentation
08:40–08:50
|
EPSC2024-139
|
ECP
|
On-site presentation
08:50–09:00
|
EPSC2024-551
|
ECP
|
On-site presentation
09:10–09:15
Q&A
09:15–09:25
|
EPSC2024-825
|
ECP
|
On-site presentation
09:25–09:35
|
EPSC2024-746
|
On-site presentation
Asteroids & Minor moons
09:35–09:45
|
EPSC2024-1174
|
ECP
|
On-site presentation
09:45–09:55
|
EPSC2024-910
|
ECP
|
On-site presentation
09:55–10:00
Q&A
Coffee break
Chairpersons: Anton Ermakov, Bart Root, Haifeng Xiao
10:30–10:40
|
EPSC2024-507
|
On-site presentation
10:40–10:55
|
EPSC2024-895
|
ECP
|
On-site presentation
10:55–11:05
Q&A
Techniques and Instruments for Planetary Geodesy
11:15–11:25
|
EPSC2024-820
|
ECP
|
On-site presentation
11:25–11:35
|
EPSC2024-787
|
On-site presentation
11:35–11:50
|
EPSC2024-641
|
On-site presentation
11:50–12:00
Q&A
Rotation & Tides
P21
|
EPSC2024-964
|
ECP
|
On-site presentation
P23
|
EPSC2024-1089
|
On-site presentation
Interior Structure & Shape
P25
|
EPSC2024-374
|
On-site presentation
P27
|
EPSC2024-570
|
ECP
|
On-site presentation
Techniques for Planetary Geodesy
P28
|
EPSC2024-785
|
ECP
|
On-site presentation
P29
|
EPSC2024-986
|
ECP
|
On-site presentation